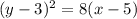Answer:
The equation of the parabola is;
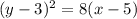
Step-by-step explanation:
Given the vertex;

and focus;

From the coordinates of the focus and the vertex we can observe that the focus and the vertex are on the same y-cordinates which means that they are on the same horizontal line.
So, the line of symmetry is perpendicular to the y axis and the parabola is an horizontal parabola.
The equation of the horizontal parabola can be derived using the equation;

Given;
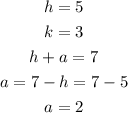
Substituting the values;
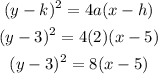
Therefore, the equation of the parabola is;