The maximum revenue will be at the x-coordinate of the vertex point of the equation
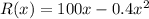
The form of the quadratic function is

Its vertex is (h, k), where
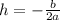
From the given function
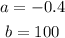
Substitute them to find the value of h
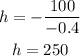
Then the x-coordinate of the maximum point of the function is 250
The number of rooms rented which produces the maximum revenue is 250 rooms
The answer is 250