Question

A 0.350 kg iron horseshoe that is initially at 465°C is dropped into a bucket containing 21.4 kgof water at 21.2°C. What is the final equilibrium temperature (in °C)? Neglect any heat transferto or from the surroundings

Answer 1

Given data

*The given mass of the iron is m_i = 0.350 kg

*The given initial temperature of the iron is T_i = 465 °C

*The given mass of the water is m_w = 21.4 kg

*The given initial temperature of the water is T = 21.2 °C

*The value of the specific heat of water is c_w = 4186J/kg.°C

*The value of the specific heat of iron is c_i = 448J/kg.°C

The total energy input from the surrounding is equal to zero. It is given as

`\begin{gathered} Q_i+Q_w=0 \\ m_ic_i\Delta T+m_wc_w\Delta T=0 \end{gathered}`

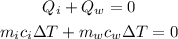
Substitute the known values in the above expression as

`\begin{gathered} m_ic_i(T_i-t)+m_wc_w(T-t)=0 \\ t=(m_wc_wT+m_ic_iT_i)/(m_wc_w+m_ic_i) \\ =21.97^0\text{ C} \end{gathered}`

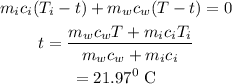
Hence, the final equilibrium temperature (in °C) is t = 21.97°C