ANSWER
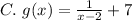
Step-by-step explanation
The given function is:
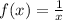
When a function is shifted vertically upward, it changes as follows:
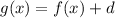
where d = number of units that it is shifted
Therefore, shifting f(x) by 7 units up will result in the following function:
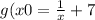
When a function is shifted horizontally to the right, it changes as follows:
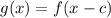
where c = number of units that it is shifted
Hence, transforming the function above by shifting it 2 units to the right will result in:

The correct answer is option C.