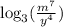Question

I somewhat understand where to begin with this equation, but am struggling to comprehend how to solve it!

Answer 1

We have the following properties of logarithms:

`\begin{gathered} \log (a\cdot b)=\log (a)+\log (b) \\ \log ((a)/(b))=\log (a)-\log (b) \\ \log (a^b)=b\cdot\log (a) \end{gathered}`

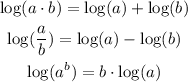
in this case we have the following expression:

`\log _3y+7\log _3m-5\log _3y`

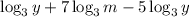
using the third property, we get:

`\log _3y+7\log _3m-5\log _3y=\log _3y+\log _3m^7-\log _3y^5`

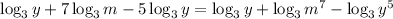
next, we can use the first property on the first two summands to get the following:

`\log _3y+\log _3m^7-\log y^5=\log _3(ym^7)-\log _3y^5`

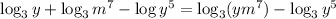
finally, using the second property, we get:

`\log _3(ym^7)-\log _3y^5=\log _3((ym^7)/(y^5))=\log _3((m^7)/(y^4))`

therefore, the expresson as a single logarithm is:

`\log _3((m^7)/(y^4))`