Question

3.Draw diagrams to show the first three modes of resonance in the following instruments (also find the frequencies of these modes if the air temperature 20 °C): a)A clarinet 75 cm long that functions acoustically like a closed air column. b)An organ pipe open at both ends that is 4.8 m long. c)A guitar string 1.3 m long with a wave speed of 66 m/s.

Answer 1

Given:

a) A clarinet 75 cm long that functions acoustically like a closed air column.

b) An organ pipe open at both ends that is 4.8 m long

c) An organ pipe open at both ends that is 4.8 m long

To find:

Draw diagrams to show the first three modes of resonance

Step-by-step explanation:

a)

A clarinet is as same as a closed pipe.

The first three resonances are,

`\begin{gathered} n=(V)/(4L) \\ V=343\text{ m/s \lparen speed of air at 20}\degree C) \\ L=75\text{ cm} \\ =0.75\text{ m} \\ \end{gathered}`

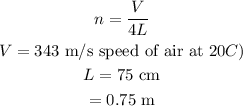
Now,

`\begin{gathered} n=(343)/(4*0.75) \\ =114.3\text{ Hz} \\ \end{gathered}`

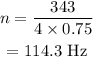
The next two resonances are,

`\begin{gathered} n_1=3n \\ =3*114.3 \\ =343\text{ Hz} \\ n_2=5*114.3 \\ =571.5\text{ Hz} \end{gathered}`

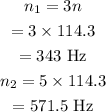
Hence, the frequencies are 114.3 Hz, 343 Hz, and 571.5 Hz.

The resonances are as shown below:

b)

For an open organ pipe:

The first three resonances are,

`\begin{gathered} n=(V)/(2L) \\ V=343\text{ m/s \lparen speed of sound in air at 20 degrees\rparen} \\ L=4.8\text{ m} \\ n=(343)/(2*4.8) \\ =35.7\text{ Hz} \\ n_1=2n \\ =71.4\text{ Hz} \\ n_2=3n \\ =107.1\text{ Hz} \end{gathered}`

The diagram is as shown below:

Hence, the frequencies are 35.7 Hz, 71.4 Hz, and 107.1 Hz.

c)

The frequencies of the first three resonances in a guitar are,

`\begin{gathered} n=(v)/(2l) \\ v=66\text{ m/s} \\ l=1.3\text{ m} \\ n=(66)/(1.3) \\ =50.8\text{ Hz} \\ n_1=2n \\ =101.6\text{ Hz} \\ n_2=3n \\ =152.4\text{ Hz} \end{gathered}`

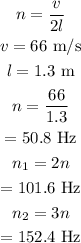
Hence, the frequencies are 50.8 Hz, 101.6 Hz, and 152.4 Hz.

The diagram of the modes are as shown below: