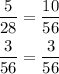Use the least common denominator to enter an equivalent fraction for ea fraction.

Question

asked Mar 24, 2023 165k views

1 Answer

Renzo Robles · Answer 1 · 2023-03-29T01:45:40+0000

Given

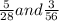
To find the LCD, we find the LCM of the denominators. Find the LCM of 28 and 56:

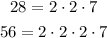
$\begin{gathered} 28=2\cdot2\cdot7 \\ 56=2\cdot2\cdot2\cdot7 \end{gathered}$

So:

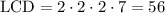
$\text{LCD}=2\cdot2\cdot2\cdot7=56$

Next, to write 5/28 as a fraction with a common denominator we will multiply the denominator and numerator by 2. This is:

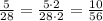
$(5)/(28)=(5\cdot2)/(28\cdot2)=(10)/(56)$

And to write 3/56 as a fraction with a common denominator we will multiply the denominator and numerator by 1. So:

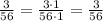
$(3)/(56)=(3\cdot1)/(56\cdot1)=(3)/(56)$

Answer:

$\begin{gathered} (5)/(28)=(10)/(56) \\ (3)/(56)=(3)/(56) \end{gathered}$