Question

A billboard of mass 150 kg hang stationary from two vertical wires. What is the tension in each of the wires?

Answer 1

Given:

Mass = 150 kg

Given that the billboard hangs from two vertical wires, let's fins the tension in each of the wires.

To find the tension in each wire, apply the formula:

`T=mg`

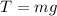
Where:

m is the mass = 150 kg

g is the acceleration due to gravity = 9.8 m/s²

Thus, we have:

`\begin{gathered} T=150*9.8 \\ \\ T=1470\text{ N} \end{gathered}`

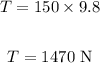
Since the total upward force (tension) is equal to the weight of the billboard and assuming the wires are of equal length, to find the length of each vertical wire let's divide the tension by 2:

`T_{\text{each}}=(1470)/(2)=735N`

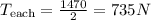
Therefore, the tension in each wire is 735 N.

ANSWER:

735 N