To answer this question, we need to know that the x- and y-intercepts are:
• The x-intercept: (5, 0). The point where the line passes through the x-axis.
,
• The y-intercept: (0, 3). The point where the line passes through the y-axis.
To find the equation of the line, we can use the two-point form equation of the line, and then we will find the standard form of the line, which is of the form:
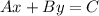
We still need to label both points:
• (5, 0) ---> x1 = 5, y1 = 0.
,
• (0, 3) ---> x2 = 0, y2 = 3.
The two-point form of the line is given by:
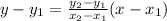
Then, substituting these values into this equation, we have:
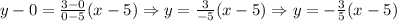
Then, we have:
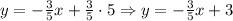
This is the slope-intercept form of the line. To find the standard form of the line, we can multiply the equation by 5 as follows:

Adding 3x to both sides of the equation, we have:
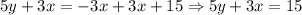
Finally, the standard form of the line is given by 3x + 5y = 15.