Question

Simplify the following expression.
`{3}^{ (11)/(5) } / {3}^{ - (9)/(5) }`

Answer 1

Simplify the expression:

`3^{(11)/(5)}/3^{-(9)/(5)}`

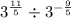
To better and easily simplify the division, we use the rule of the reciprocal. Dividing by an expression is equivalent to multiplying by its reciprocal, that is, multiplying by the same expression but changing the sing of the exponent as follows:

`3^{(11)/(5)}/3^{-(9)/(5)}=3^{(11)/(5)+(9)/(5)}`

Operating:

`3^{(11)/(5)}/3^{-(9)/(5)}=3^{(20)/(5)}`

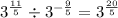
Simplifying:

`3^{(11)/(5)}/3^{-(9)/(5)}=3^4=81`

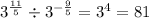
Answer: 81