Question

Suppose you are required to design a rectangu-lar medicine container such that the ratio of thelength to the width to the height is 5:3:2,and the volume of the container equals120 cm?, how would you determine the dimen-sions (length, width, height) of this container?

Answer 1

For this case we know that we need to design a rectangular container and we have the following ratio:

lenght:width:height (5:3:2)

We also know that the total volume is given by 120 cm^3.

And we want to determine the dimensions of the container.

Step 1: Formula for the volume

Since we have a rectangular container the volume would be given by:

`V=l\cdot w\cdot h`

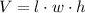
Where l= legth, w= width and h=height

Step 2 : Set up the formulas to use

If we select a dimension fix for example the lenght we can do the following:

`V=l((3)/(5)l)((2)/(5)l)`

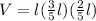
The reason of this is because we have that:

`(l)/(w)=(5)/(3)\rightarrow w=(3)/(5)l`
`(l)/(h)=(5)/(2)\rightarrow h=(2)/(5)l`

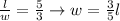
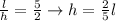
Using the formula of the volume with l we can do this:

Step 3: Solving for the answers

And solving for l we got:

And we can find the other dimensions like this: