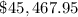Question

LUMP SUM DEPOSIT Parents expect to need $160,000 for College when their baby grows up. They will deposit a lump sum into an account that pays 7% interest compounded TWICE A MONTH for 18 years. They will leave the money alone for the full 18 years. How much should they deposit now? (I highlighted TWICE A MONTH because we are used to examples where the compounding happens 12 times a year; here, the compounding will occur 24 times in a year.)

Answer 1

They should deposit $45,467.95 now.

`\text{ \$}45,467.95`

Step-by-step explanation:

The formula for calculating the future value of compound interest can be written as;

`A=P(1+(r)/(n))^(nt)`

Where;

A = Future amount

P = Principal (initial amount)

r = Interest rate (decimal)

n = number of times the interest is compounded per unit time "t"

t = time

Making the Principal (P) the subject of formula in the formula above we have;

`P=(A)/((1+(r)/(n))^(nt))`

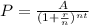
Given;

A = Future amount = $160,000

r = Interest rate (decimal) = 7% = 0.07

n = number of times the interest is compounded per unit time "t" = 2(12) = 24 times per year

(Twice in a month times 12 months in a year.)

t = 18 years

substituting the given values into the formula above, we have;

`\begin{gathered} P=(160,000)/((1+(0.07)/(24))^(24(18)))=(160,000)/((1+(0.07)/(24))^(432)) \\ P=\text{ \$}45,467.95 \end{gathered}`

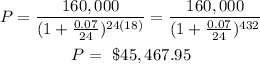
Therefore, They should deposit $45,467.95 now.

`\text{ \$}45,467.95`