Question

I got this wrong so please help me solve it, the answers in the gray boxes are the correct ones

Answer 1

75.8 miles per hour, 83.3°

Explanation:

Given:

Plane velocity is 350 mi/hr N70E° (90 - 70 = 20°)

Ground velocity is 390 mi/hr, 30°

The ground velocity is the magnitude V + W, just like this:

`\begin{gathered} V+W=390\cdot\cos 30i+390\cdot\sin 30j \\ V+W=337.7i+195j \end{gathered}`

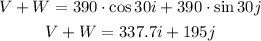
We know that for V it would be:

`\begin{gathered} V=350\cdot\cos 20i+350\cdot\sin 20j \\ V=328.9i+119.7j \end{gathered}`

Therefore:

`\begin{gathered} V+W-V=337.7i+195j-328.9i-119.7j \\ W=8.8i+75.3j \end{gathered}`

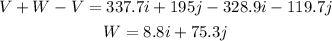
We calculate the magnitude using the norm of the vector, like this:

`\begin{gathered} |W|=√(\left(8.8\right)^2+\left(75.3\right)^2) \\ |W|=\sqrt[]{77.4+5670.9} \\ |W|=\sqrt[]{5747.53} \\ |W|=75.8 \end{gathered}`

The speed of wind is 75.8 miles per hour

We calculate the direction of the angle as follows:

`\begin{gathered} \tan \theta=(y)/(x) \\ \theta=\tan ^(-1)\mleft((75.3)/(8.8)\mright) \\ \theta=83.3\degree \end{gathered}`

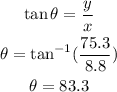
The direction angle of the wind is 83.3°