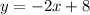Question

Find the equation of a line that goes through the point ( 2,4 ) and is parallel to the line y = - 2x + 17Select one:a.y = - 2x + 8b.y = 2x + 4c.y = 2x + 17d.y = - 2x + 1

Answer 1

Answer: a.

`y=-2x+8`

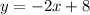
Step-by-step explanation

Given

• Goes through the point ( 2,4 ).

,

• Parallel to the line y = - 2x + 17.

Procedure

We can find the equation in the slope-intercept form:

`y=mx+b`

where m is the slope and b is the y-intercept.

Considering that the slopes of parallel lines are the same, then our slope is m = –2:

`y=-2x+b`

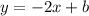
Next, using the point given ( 2,4 ) we can find b by replacing the values in y and x, respectively, and solving for b:

`4=-2(2)+b`
`4=-4+b`
`4+4=b`
`b=8`

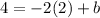
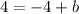
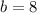
Finally, replacing our results in the slope-intercept form we get:

`y=-2x+8`