We know that the limit
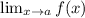
exists if and only if:
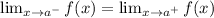
Let's calculate the left sided limit, for this limit we will use the first expression:

Let's find the right sided limit, for this limit we will use the second expression:
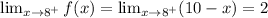
We notice that these limits are not equal which means that the limit does not exist.
We can visualize this with the graph of the function which is shown below:
From the graph we notice that as we approach eight the value of the function is not the same for each side which means that the limit does not exist.