Question

Geometry :)


pls help! thank you soo much :)
( image attached)

Answer 1

m∠ABC = 81°

m∠FEC = 90°

Explanation:

A median of a triangle is a line segment from the vertex to the mid-point of the side opposite that vertex.

If AB ≅ BC then triangle ABC is an isosceles triangle.

Therefore, if BE is the median of triangle ABC, then m∠ABE ≅ m∠CBE.

Therefore, the measure of ∠ABC is twice the measure of ∠ABE.

First, convert the given measure of ∠ABE into decimal degrees.

`\boxed{\textsf{Decimal degrees}=\sf Degrees + (Minutes)/(60)+ (Seconds)/(3600)}`

`\begin{aligned}\implies \angle ABE=40^(\circ)30'&=40+(30)/(60)+(0)/(3600)\\&=40+0.5+0\\&=40.5^(\circ)\end{aligned}`

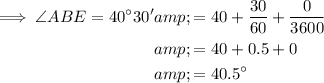
Therefore, m∠ABE = 40.5°.

If m∠ABC is twice the measure of ∠ABE:

`\begin{aligned}\implies m \angle ABC&=2 * \angle ABE\\&=2 * 40.5^(\circ)\\&=81^(\circ)\end{aligned}`

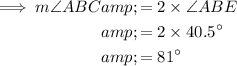
As triangle ABC is an isosceles triangle where AB≅BC, the median BE is perpendicular to AC. Therefore, m∠FEC = 90°.