The formula of the partial sum of the first n terms of a geometric series is:
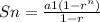
In this case, a1 is 6 (the first term of the series) and r is 1/4 (the base). By replacing 16 for n and the rest of the values we should get:
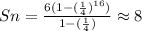
Then, the sum of the given geometric series is 8