Solution
Step 1:
The figure below illustrate the given informations:
Step 2:
Let the length of the wire be l.
Use the Pythagoras theorem to find the length of the wire:

Step 3
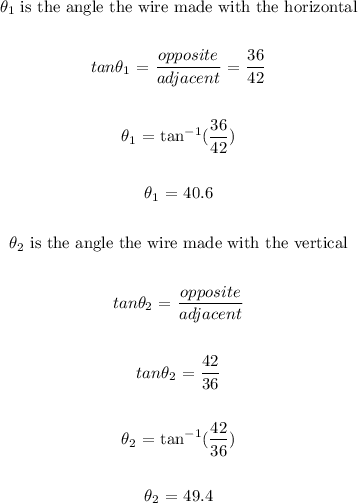
Final answer
The length of the wire is 55.3 meters
The angle between the tower and the wire is 49.4