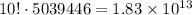Question

DJ is making a playlist for a radio show, he was trying to decide what 10 songs to play and in what order they should be played. if he has his choices narrowed down to 7 Blues, 7disco, 5 pop, and 7 Reggie songs, and he wants to play no more than four reggae songs how many different playlist are possible? Express the answer as a scientific notation rounded to the hundredth place

Answer 1

Since the DJ doesn't want to play more than 4 Reggae songs he can chooses 0, 1, 2, 3 or 4 reggae songs.

Case 1. The DJ chooses 0 Raggae song.

In this case all the songs are selected from the 19 possible songs of different genre, using combinations defined by:

`_nC_k=(n!)/(k!(n-k)!)`

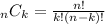
We have a total of:

`_(19)C_(10)\cdot_7C_0=92378`

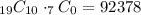
Case 2. The DJ chooses 1 Raggae song.

In this case 9 songs are selected from the 19 songs of other genre then the total possibilities are:

`_(19)C_9\cdot_7C_1=646646`

Case 3. The DJ chooses 2 Raggae songs.

In this case 8 songs are selected from the 19 songs of other genre then the total possibilities are:

`_(19)C_8\cdot_7C_2=1587222`

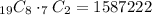
Case 4. The DJ chooses 3 Raggae songs.

In this case 7 songs are selected from the 19 songs of other genre then the total possibilities are:

`_(19)C_7\cdot_7C_3=1763580`

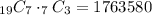
Case 5. The DJ chooses 4 Raggae songs.

In this case 6 songs are selected from the 19 songs of other genre then the total possibilities are:

`_(19)C_6\cdot_7C_4=949620`

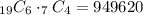
So the total possible ways of choosing the playlist is 5039446.

Now the playlist can be arrange in 10! different ways, hence the are different playlists:

`10!\cdot5039446=1.83*10^(13)`