Answer:
To figure out the mean, we will use the formula below
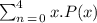
when x=0

When x=1
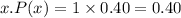
when x=2
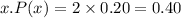
When x=3
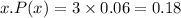
When x= 4
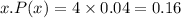
Hence,
The mean of the distribution will be
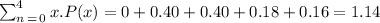
Hence,
The mean is

To figure out the standar deviation, we will use the formula below

when x=0
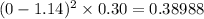
when x= 1

when x=2
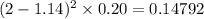
When x=3

When x=4
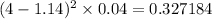
Add them up and find the square root
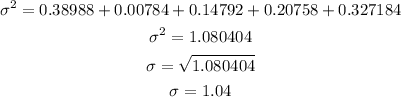
Hence,
The mean = 1.14 and the standard deviation is =1.04
OPTION A is the right answer