Question

PLEASE HELP!!!Describe the sequence of transformations used to produce the graph each function.

Answer 1

We are asked to describe sequence of transformations used to produce the graph for each function.​

First of all, let us understand transformation rules for functions.

Vertical Translation:

`\begin{gathered} f(x)+d\Rightarrow\text{vertical translation up by d units} \\ f(x)-d\Rightarrow\text{vertical translation down by d units} \end{gathered}`

Horizontal Translation:

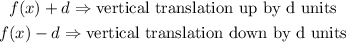
`\begin{gathered} f(x+c)\Rightarrow\text{horizontal translation left by c units} \\ f(x-c)\Rightarrow\text{horizontal translation right by c units} \end{gathered}`

Reflection:

`\begin{gathered} -f(x)\Rightarrow\text{ reflection over x-axis} \\ f(-x)\Rightarrow\text{ reflection over y-axis} \end{gathered}`

Dilation:

`\begin{gathered} a\cdot f(x)\Rightarrow\text vertical stretch for a|>1 \\ a\cdot f(x)\Rightarrow\text{ vertical compression for }0<|a|<1 \end{gathered}`

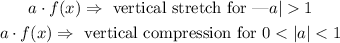
Function 14:

`y=-\sqrt[]{x+4}+1`

As you can see,

it is reflected over the x-axis

(x+4) means translated left by 4 units

Also, +1 translated up by 1 unit

Function 15:

`y=(1)/(4)|x+5|-4`

As you can see,

It is vertically compressed by 1/4

(x+5) means translated left by 5 units

Also, -4 means translated down by 4 units

Function 16:

`y=\sqrt[3]{-x}+5`

As you can see,

it is reflected over the y-axis since f(-x)

+5 means translated up by 5 unit

Function 17:

`y=-3(x+2)^2`

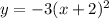
As you can see,

it is reflected over the x-axis

(x+2) means translated left by 2 units

Also, It is vertically stretched by 3

Function 18:

`y=(1)/(2)(x-4)^3-1`

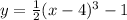
As you can see,

(x - 4) means translated right by 2 units

-1 means translated down by 1 unit

Also, It is vertically compressed by 1/2