The graph of cost vs number of text messages seem to have a flat fee for up to 800 messages (although the numbers are not specified in the x-axis).
A. f(1400) = 60 means that the cost of sending 1400 text messages is 60 dollars.
B. It seems to be a plan with 800 messages included for 30 dollars. For any additional message, there is a cost per message.
This cause the graph to increase with a constant rate. The slope of this line is the cost per additional message.
We can calculate the slope using the known points f(800)=30 and f(1400)=60:
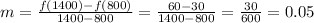
The slope is m=0.05.
We can conclude that any additional message, above the 800 messages included, cost 0.05 dollars.