Question

Find the x- and y-intercepts of the graph of 3x + 10y = 33. State each answer as an integer or an improper fraction in simplest form.

Answer 1

Given:

The graph of a straight line is given as,

`3x+10y=33`

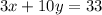
The objective is to find the x-intercept and y-intercept of the graph.

Step-by-step explanation:

To find x-intercept:

Substitute y = 0 in the given equation.

`\begin{gathered} 3x+10(0)=33 \\ x=(33)/(3) \\ x=11 \end{gathered}`

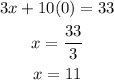
Thus, the coordinate is (11,0).

To find y-intercept:

Substitute x = 0 in the given equation.

`\begin{gathered} 3(0)+10y=33 \\ y=(33)/(10) \\ y=3.3 \end{gathered}`

Thus, the coordinate is (0,3.3).

Hence, the x-intercept is (11,0) and y-intercept is (0,3.3).