Question

Solve the exponential equation. Round your answer to the nearest hundredth:20^r = 56

Answer 1

For this problem, we are given a certain exponential equation. We need to solve it for "r".

The equation is:

`20^r=56`

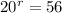
The first step is to apply a logarithm on both sides:

`\begin{gathered} \log20^r=\log56 \\ \end{gathered}`

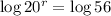
Now we can use the exponent rule to isolate the "r"

`\begin{gathered} r\log20=\log56\\ \\ r=(\log56)/(\log20)=1.34369 \end{gathered}`

The real solution for this problem is 1.34.