Question

A) P(t) = ___B) What population do you predict for the year 2000?Predicted population in the year 2000= ___ million people C) What is the doubling time?Doubling time= ____years.

Answer 1

Given:

The number of people in 1980 is 15 million.

The number of people in 1990 is 60 million.

Let t be the number of years.

The difference between 1990 and 1980 is 10 years.

Consider the general exponential equation

`P(t)=ae^(bt)`

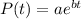
Substitute t=0 and P(0)=15, we get

`15=ae^(b(0))`
`a=15`

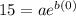
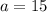
Substitute a=15 in the general equation, we get

`P(t)=15e^(bt)`

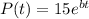
Substitute t=10 and P(10)=60, we get

`60=15e^(b(10))`

`(60)/(15)=e^(10b)`

`4=e^(10b)`

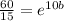
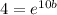
Taking log on both sides, we get

`In(4)=10b`

`1.38629=10b`

`b=(1.38629)/(10)=0.138629`

`b=0.139`

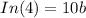
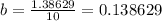
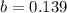
Substitute b=0.139 and a=15 in the general equation, we get

`P(t)=15e^(0.139(t))`

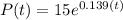
Hence the exponential equation is

`P(t)=15e^(0.139(t))`

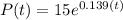
In the year 2000, t=20.

Substitute t=20 in P(t), we get

`P(20)=15e^(0.139(20))`

`P(20)=15e^(2.78)`

`P(20)=241.78`

`P(20)=242`

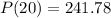
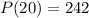
In the year 2000, the predicted population is 242 million.

The doubling time is the time when the population is double.

Substitute P(t)=30 to find the doubling time.

`30=15e^(0.139(t))`

`(30)/(15)=e^(0.139(t))`

`2=e^(0.139(t))`

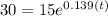
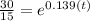
Taking log on both sides, we get

`In(2)=0.139\mleft(t\mright)^{}`

`(0.6931)/(0.139)=t`
`t=4.98`
`t=5\text{ years}`

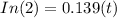
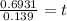
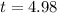
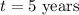
The doubling time is 5 years.