Question

A steel plug has a diameter of 5cm at 30°C.At what temperature will it fit exactly into a hole of constant diameter of 4.997cm?(coefficient of linear expansion of steel is 11×10^-6°C^-1)

Answer 1

By the definition of linear thermal expansion:

`\begin{gathered} \Delta L=\alpha L_0\Delta T \\ L-L_0=\alpha L_0(T-T_0) \end{gathered}`

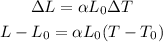
Here, L_0=4.997 cm=0.04997 m is the initial diameter of the steel plug at some temperature T_0, L=5 cm=0.05 m is the diameter of steel plug at T=30°C, α=11*10^(-6) °C^(-1) is the linear expansion of steel.

Rearranging the above equation to get an expression for T_0,

`\begin{gathered} T-T_0=(L-L_0)/(\alpha L_0) \\ T_0=T-(L-L_0)/(\alpha L_0) \end{gathered}`

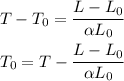
Substituting all known values,

`\begin{gathered} T_0=(30^(\circ)C)-\frac{(0.05\text{ m})-(0.04997\text{ m})}{(11*10^(-6\circ)C^(-1))*(0.04997\text{ m})} \\ =-24.58\degree C \end{gathered}`

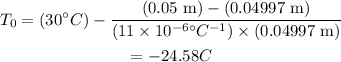
Therefore, at -24.58°C the stell plug will fit exactly into a hole of the constant diameter of 4.997 cm.