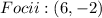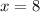Question

Find the focus and directrix: (y + 2)2 = –4(x – 7).

Answer 1

Given:

`(y+2)^2=-4(x-7)`

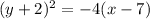
Required:

We need to find the focus and directrix.

Step-by-step explanation:

Consider the parabola equation.

`(y-k)^2=a(x-h)`

Compare this equation with the given equation.

`We\text{ get }k=-2,\text{ h=7 and a =-4.}`

Consider the formula to find the focii.

`Focii:(h+(a)/(4),k)`

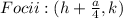
Substitute h=7, a=-4, and k=-2 in the formula.

`Focii:(7+(-4)/(4),-2)`

`Focii:(7-1,-2)`

`Focii:(6,-2)`

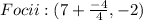
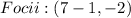
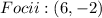
Consider the formula to find directrix.

`x=h-(a)/(4)`

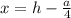
Substitute h=7, and a=-4 in the formula.

`x=7-((-4))/(4)`

`x=7-(-1)`
`x=8`

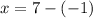
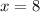
Final answer:

`Focii:(6,-2)`

`x=8`