Question

Please look for the question in the picture.

Answer 1

Hello There!

Question:-

`\sf \longmapsto1/4x-3/5=1(2)/(5)`

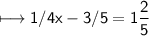
We need to find the value of x.

Solution:-

Convert
`(2)/(5)` to improper fraction:-

`\sf \longmapsto(1)/(4) x + ( - 3)/(5) = (7)/(5)`

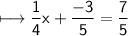
Add 3/5 to both sides:-

`\sf \longmapsto \: (1)/(4) x + ( - 3)/(5) + \red {(3)/(5)} = (7)/(5) + \red{(3)/(5)}`

On Simplification:-

`\sf \longmapsto \: (1)/(4) x + ( 3)/(5) - (3)/(5) = (7 + 3)/(5)`

`\sf \longmapsto \: (1)/(4) x - 0 = \cancel (10)/(5)`

`\sf \longmapsto (1)/(4) x = 2`

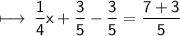
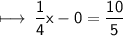
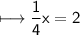
Multiply both sides by 4 :-

`\sf \longmapsto \: \red4 * \bigg( (1)/(4) x \bigg) = \red 4 * 2`

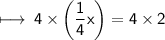
On Simplification:-

`\sf \longmapsto \: \cancel 4 * (1)/( \cancel4)x = 4 * 2`

`\sf \longmapsto \: x = 8`

______________________________________

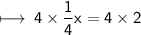
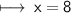
Henceforth,The value of x is :-

`\boxed{ \huge\bf \: x = 8}`

________________________________

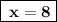
Please let me know if you have any questions.

~MisterBrian

Answer 2

x = 8

Explanation:

`(1)/(4) x-(3)/(5) =1(2)/(5)`

`(1x)/(4) -(3)/(5) =(7)/(5)`

`(1x)/(4) =(7)/(5) +(3)/(5) \\`

`(1x)/(4) =(10)/(5)`

Use cross multiplication.

`5x=4*10`

`5x=40`

`(5x)/(5)=(40)/(5)`

`x=8`

Hope this helps you.

Let me know if you have any other questions :-)