Question

Solve this system of equations byusing the elimination method.2x + 4y = 16-2x – 3y = -6([?], [])The ordered pair of solutionsis written in the format (x, y).

Answer 1

Given:

`\begin{gathered} 2x\text{ + 4y = 16} \\ -2x\text{ - 3y = -6} \end{gathered}`

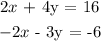
Step 1: Multiply each equation by a suitable number so that the two equations have the same leading coefficient

Since the leading coefficient of x of the two equations are the same number, we can skip this part

Step 2: Add the second equation to the first.

`\begin{gathered} (2x\text{ + 4y})\text{ + }(-2x\text{ -3y})\text{ = 16 +}(\text{- 6}) \\ 2x\text{ - 2x + 4y - 3y = 16 -6} \\ y\text{ = 10} \end{gathered}`

Step 3: Substitute the value of y into any of the equation and solve for x

`\begin{gathered} 2x\text{ + 4y = 16} \\ 2x\text{ + 4}*10\text{ = 16} \\ 2x\text{ + 40 = 16} \\ Collect\text{ like terms} \\ 2x\text{ = 16- 40} \\ 2x\text{ = -24} \\ Divide\text{ both sides by 2} \\ x\text{ = -12} \end{gathered}`

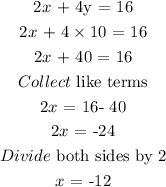
Hence, the ordered pair of solution is (-12, 10)