Question

Find the vertex of f(x)=5x^2+40x-3

Answer 1

From the question given:

f(x) = 5x² + 40x - 3

comparing the above with ax² + bx + c

a = 5

b = 40

c=-3

The x - axis of the vertex can be gotten by simply finding the value of -b/2a

`x=(-b)/(2a)`

`=(-10)/(2(5))=(-40)/(10)=\text{ -}4`

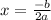
To get the y-axis of the vertex, simply substitute the value of x=-4 into the function given

That is ;

`f(-1)=5(-4)^2+\text{ 40(-4) - 3}`
`=5(16)\text{ - 160 - 3}`

`=80\text{ - 160 - 3}`
`=\text{ -83}`

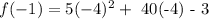
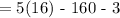
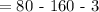
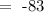
The function has a vertex at: (-4, -83)