Question

Leo drew a line that is perpendicular to the line shown on the grid and passes through point (F, G). Which of the following is the equation of Leo's line? Image and answers are attached

Answer 1

To answer the question, we will calculate the slope of the line given.

The formula to calculate the slope of a line is given to be:

`m=(y_2-y_1)/(x_2-x_1)`

We can pick any 2 points on the graph as shown below:

Hence, we have the coordinates:

`\begin{gathered} (x_1,y_1)=(1,1) \\ (x_2,y_2)=(2,4) \end{gathered}`

Therefore, the slope is calculated to be:

`m=(4-1)/(2-1)=3`

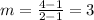
Recall that perpendicular lines have slopes that are inverse reciprocals of each other.

Therefore, the slope of the line perpendicular to the one in the graph is:

`m=-(1)/(3)`

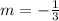
Given a point and the slope of a line, the point-slope formula can be used to write out the equation of the line:

`y-y_1=m(x-x_1)`

Given the point (F, G) and the slope -1/3, the equation of the perpendicular line will be:

`y-G=-(1)/(3)(x-F)`

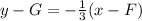
The SECOND OPTION is correct.