Question

create 2 liner equations in the form y=mx+bthe lines are perpendicular to eachother both lines pass through the point (3,-5)

Answer 1

The linear equations are to be written in the form:

`y=mx+b`

Let the lines be:

`\begin{gathered} \text{Line 1} \\ y=m_1x+b_1 \\ \text{Line 2} \\ y=m_2x+b_2 \end{gathered}`

Since both lines are perpendicular, we have that:

`m_1=-\frac{1}{m_2_{}}`

Therefore, the equation for Line 1 becomes:

`y=-(x)/(m_2)+b_1`

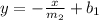
Assuming the slope m₂ is 2, we have the equations to be:

`\begin{gathered} \text{Line 1} \\ y=-(x)/(2)+b_1 \\ \text{Line 2} \\ y=2x+b_2 \end{gathered}`

We can get the values of b₁ and b₂ by substituting the values of x and y given to be the ordered pair (3, -5):

`\begin{gathered} \text{Line 1} \\ -5=-(3)/(2)+b_1 \\ b_1=-5+(3)/(2) \\ b_1=-3.5 \end{gathered}`

and

`\begin{gathered} \text{Line 2} \\ -5=2(3)+b_2 \\ b_2=-5-6 \\ b_2=-11 \end{gathered}`

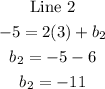
Therefore, the 2 linear equations can be:

`\begin{gathered} y=-(x)/(2)-3.5\text{ ---------(1)} \\ y=2x-11\text{ ----------(2)} \end{gathered}`

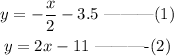
The graph of the 2 equations are shown below: