Question

If all men had identical body types, their weight would vary directly as the cube of theirheight. The tallest person reached a record height of 8 feet 11 inches (107 inches) beforehis death at age 22. If a man who is 5 feet 10 inches tall (70 inches) with the same bodytype as the tallest person weighs 190 pounds, what was the tallest person's weight shortlybefore his death?The tallest person's weight was aboutbefore his death.(Simplify your answer. Round to the nearest whole number as needed.)

Answer 1

`\because w\propto h^3`

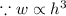
The weight is directly proportioned with the cube of the height

w is the weight

h is the height

∵ The height of the tallest man is 107 inches

∵ The man has a height of 70 inches and a weight of 190 pounds

By using the proportion given above

`\because(w_1)/(w_2)=((h_1)^3)/((h_2)^3)`

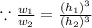
w1 = ?, w2 = 190

h1 = 107, h2 = 70

Substitute them in the rule above to find w1

`\because(w_1)/(190)=((107)^3)/((70)^3)`

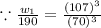
By using cross multiplication

`\therefore w_1*343000=190*1225043`
`\therefore343000w_1=232758170`

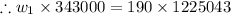
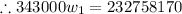
Divide both sides by 343000 to find w1

`\therefore w_1=678.5952478`

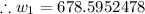
Round it to the nearest whole number

`\therefore w_1=679\text{ pounds}`

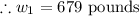
The tallest person's weight was about 679 pounds