The z-score can be found the following formula:
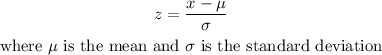
x is the value we want to find the z-score, we need to do this first as follows:
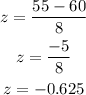
Thus, the find the proportion of individuals that score more than 55 points, we need to find the probability of z>-0.625. It can be written as:
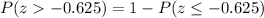
In a standard normal table, we can search the probability of z<=-0.625, it is:
0.26599.
By replacing this value in the formula we obtain:
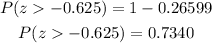
The proportion of individuals that score more than 55 points on this test is 73.4% of the total number of individuals.