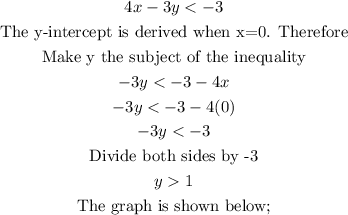
Number (2)

Observe that for the first inequality, the y intercept is 1, that is, the graph crosses the y axis at the point where y = 1. Hence all values of the inequality lies at the point where y > 1 (as shown by the shaded region)
Similarly, for the second inequality, the y intercept is -2. Hence all the values of the inequality lies at the shaded region where all values of y is greater than -2