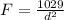Given:
F varies inversely with d²
So,
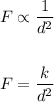
Where (k) is the proportionality constant
We will find the value of (k) using the given condition
When F=21, d= 7
Substitute with (F) and (d):
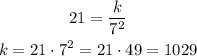
So, the answer will be:
The general formula to describe the variation is: