Question

From the top of a light house 44m above the sea, the angle of depression of a buoy is 18 degrees 30’. Find the horizontal distance to the buoy?

Answer 1

131.5m

Step-by-step explanation:

Let's go ahead and sketch the given problem;

Let's go ahead and convert the angle of depression to degrees only as seen below;

`18\text{ degr}ees\text{ 30'}=18^(\circ)+(30)/(60)=18+0.5=18.5^(\circ)`

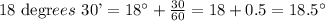
We can go ahead and solve for x which is the horizontal distance between the lighthouse and the buoy as seen in the above image;

`\begin{gathered} \tan 18.5=(44)/(x) \\ x\tan 18.5=44 \\ x=(44)/(\tan 18.5) \\ x=131.5m \end{gathered}`