Question

The time needed to paint a fence varies directly with the length of the fence and indirectly with the number of painters. If it takes five hours and 30 minutes to paint a 150 feet fence with three painters, how long will it take seven painters to paint 510 feet of fence? (DO NOT ROUND THE VALUE OF K)

Answer 1

Denote by t the time we ned to paint a fence, by l the length of the fence and by n the number of painters. Then, we have the next equation

`t=k(l)/(n)`

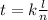
It takes 5 hours and 30 minutes, that is

`5(60)+30=330\text{ minutes}`

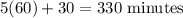
to paint a l=150 feet fence with n=3 painters, then

`\begin{gathered} 330=k(150)/(3) \\ k=(3*330)/(150) \\ k=(33)/(5) \end{gathered}`

So, if we have a l=510 feet fence and n=7 painters, then we will need

`\begin{gathered} t=(33)/(5)\cdot(510)/(7) \\ t=480.85\text{ minutes} \end{gathered}`

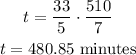
That is, approximately 8 hours.