Question

Solve the system analytically. If the equations are dependent, write the solution set in terms of the variable z. Hint, let 1/x=t,1/y=u and 1/z=w.

Answer 1

x=4, y=8 and z=1

Step-by-step explanation:

Given the system of equations:

`\begin{gathered} (3)/(x)+(2)/(y)-(2)/(z)=-1 \\ (6)/(x)-(12)/(y)+(5)/(z)=5 \\ (7)/(x)+(2)/(y)-(1)/(z)=1 \end{gathered}`

Making the substitutions: 1/x=t,1/y=u and 1/z=w., we have:

`\begin{gathered} 3t+2u-2w=-1\ldots(1) \\ 6t-12u+5w=5\ldots(2) \\ 7t+2u-w=1\ldots(3) \end{gathered}`

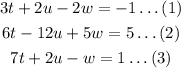
From the third equation:

`w=7t+2u-1`

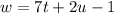
Substitute w into the first and second equations:

First Equation

`\begin{gathered} 3t+2u-2w=-1 \\ 3t+2u-2(7t+2u-1)=-1 \\ 3t+2u-14t-4u+2=-1 \\ -11t-2u=-3 \\ 11t+2u=3\ldots(4) \end{gathered}`

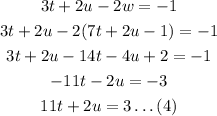
Second Equation

`\begin{gathered} 6t-12u+5w=5 \\ 6t-12u+5(7t+2u-1)=5 \\ 6t-12u+35t+10u-5=5 \\ 41t-2u=10\ldots(5) \end{gathered}`

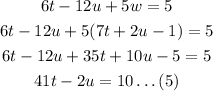
We then solve equations 4 and 5 simultaneously:

`\begin{gathered} 11t+2u=3 \\ 41t-2u=10 \\ \text{Add} \\ 52t=13 \\ t=(13)/(52) \\ t=(1)/(4) \end{gathered}`

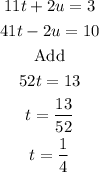
Substitute t to solve for u.

`\begin{gathered} 11t+2u=3 \\ (11)/(4)+2u=3 \\ 2u=3-(11)/(4) \\ 2u=(1)/(4) \\ u=(1)/(8) \end{gathered}`

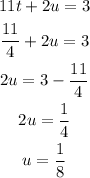
Recall that w=7t+2u-1:

`\begin{gathered} w=7((1)/(4))+2((1)/(8))-1 \\ =(7)/(4)+(2)/(8)-1 \\ w=1 \end{gathered}`

Therefore, we have that:

`\begin{gathered} (1)/(x)=(1)/(4)\implies x=4 \\ (1)/(y)=(1)/(8)\implies y=8 \\ (1)/(z)=1\implies z=1 \end{gathered}`

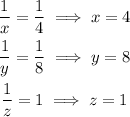
The solution to the system of equations is:

`x=4,y=8\text{ and z=1}`

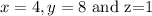
There is only one solution. The solution set is (4,8,1).