Lets call A the number of adults and S the number of students in the game.
As 200 tickets were sold, and this is the sum of students and adults, we can write:

As the amount collected ($495) is the sum of the tickets purchased by adults and the tickets purchased by students, and the tickets purchased are equal to the price multiplied by the number of tickets, we cann write:
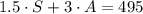
With the information for the first equation, we can replace in the second equation and solve:

Then, solving the first equation, we get;
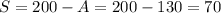
There were sold 70 student tickets and 130 adult tickets.