We have that the volume of the cone and the cylinder are the same, and the heights are related as:

It gives us the equation, using the formula of each volume:
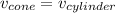

If we reply the relation of heights:

We clear the radius of cylinder as:
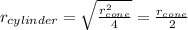
As the system of the equation gives us only the relations we need to see in the answers which option complies with the relations.
The only measure that compliest the radio relation is:
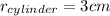
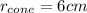
To obtain the height we need to do the same as we do with the radius.


Then we can conlude:
The height of the cone is 15 cm.
The height of the cylinder is 20 cm.
The radius of the cone is 6 cm.
The radius of the cylinder is 3 cm.The