Given:
Amount needed = $12,300
Time = 4 years
Interest = 6% ==> 0.06
Compounded annually.
Let's find the present value.
To find the present value, apply the compounded interest formula:
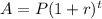
Where:
A is the amount needed = $12,300
r is the interest rate = 0.06
t is the time = 4 years
P is the principa or the present value.
Ipput values into the formula and solve for P.
We have:
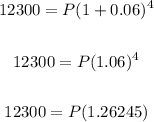
Divide both sides by 1.26245:

Therefore, the present value is $9742.75
ANSWER:
$9742.75