Question

I need help my son doesn't know how to do this

Answer 1

Required: To find x,y and z

Finding y

From the diagram 50 degrees and 5y are equal because they are corresponding angles (on same position on parallel lines)

`\begin{gathered} 5y\text{ = 50}\degree \\ \text{Dividing both sides by 5} \\ y=(50)/(5) \\ y=\text{ 10}\degree \end{gathered}`

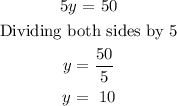
Finding z

Considering the parallel line under

130 degrees and 10z are adjacent angles ( They add up to 180 degrees)

`\begin{gathered} 130\degree\text{ + 10z = 180}\degree \\ 10z=\text{ 180}\degree-130\degree \\ 10z=\text{ 50}\degree \\ \text{Dividing both sides by 5} \\ z=\text{ 5}\degree \end{gathered}`

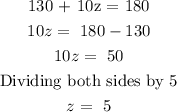
Finding x

Considering the parallel line under

The sum of all the angles on the line is 180 degrees

`\begin{gathered} 10z\text{ + x + 5y = 180}\degree \\ 10(5)\text{ + x + 5}(10)\text{ = 180}\degree \\ 50\degree\text{ + x + 50}\degree\text{ = 180}\degree \\ x=\text{ 180}\degree-\text{ 50}\degree-50\degree \\ x=\text{ 80}\degree \end{gathered}`

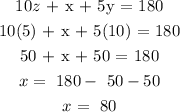
Final answers:

x= 80 degrees

y= 10 degrees

z= 5 degrees