Question

Andrea, Isla and Paulo share some money in the ratio 3:2:5The total amount of money that Isla and Pauli receive is £76 more that the amount of money that Andrea’s receives Andreas buys a video game for £48.50 with some of his share of the money Work out how much money Andrea has left from his share of the money when he has bought the game

Answer 1

Andrea has £8.50 left

Step-by-step explanation:

Ratio of Andrea, Isla, Paula = 3:2:5

Total ratio = 3 + 2 + 5 = 10

let the total amount all 3 shared = y

fraction of money received by Andrea = 3/10

fraction of money received by Isla = 2/10

fraction of money received by Paulo = 5/10

Amount received by Andrea = 3/10 × y

Amount received by Isla = 2/10 × y

Amount received by Paulo = 5/10 × y

Total amount received from Isla and Paulo = 76 more than amount received by Andrea

To make it easy, let Isla = I, Paulo = P, Andrea = A

`\begin{gathered} I+P=76+A \\ \text{from equation above},\text{ we make A the subject of formula:} \\ I\text{ + P - 76 = A } \\ I\text{ + P - 76 = }(3y)/(10)\text{ }\ldots(1) \end{gathered}`

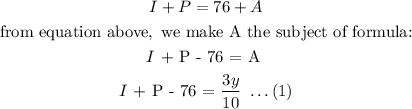
Amount received by Isla + Amount received by Paulo = 2/10 × y + 5/10 × y

`\begin{gathered} I\text{ + P = }(2y)/(10)\text{ + }(5y)/(10) \\ I\text{ + P = }\frac{7y}{10\text{ }}\text{ }\ldots\text{ (2)} \end{gathered}`

subtract equation (1) from (2):

`\begin{gathered} I\text{ - I + P - P + 0 - 76 = }(7y)/(10)\text{ - }(3y)/(10) \\ 0\text{ - (-76) = }\frac{7y\text{ - 3y}}{10} \\ 76\text{ = }(4y)/(10) \\ 76(10)\text{ = 4y} \\ y\text{ = }(760)/(4) \\ y\text{ = 190} \end{gathered}`

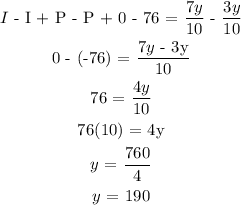
The total amount Andrea, Isla and Paulo shared = £190

Andrea spent £48.50 from his share of his money. We need to find the amount left

Amount Andrea received = 3/10 × y

Amount Andrea received = 0.3 × 190 = £57

Amount left = Andrea's amount - cost of game

Amount left = 57 - 48.5

Amount left = 8.50

Hence, Andrea has £8.50 left