ANSWER:
6 cm
Explanation:
When a triangle has the measurements 30-60-90, it has the following form (depending on the shortest leg):
Confirmed by the Pythagorean Theorem
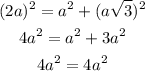
Therefore:
If the value of a is equal to 3, the hypotenuse would be:
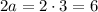
The value of the hypotenuse is 6 cm