Answer:
The equation of the line in slope-intercept form is:
y = x - 3
Step-by-step explanation:
Given the points (3, 0) and (5, 2)
The equation of a line in slope-intercept form is:
y = mx + b .....................................................(1)
Where m is the slope and b the y-intercept
To obtain the slope, we use the formula:
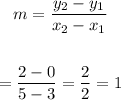
Using the obtained m = 1, the equation now becomes
y = (1)x + b
OR
y = x + b ............................................................(2)
Using either of the given points (say (3, 0)), we have x = 3 and y = 0.
Substitute these into equation (2), we have:
0 = 3 + b
b = 0 - 3 = -3
Therefore, the required equation of the line in slope-intercept form is:
y = x - 3