We are given the following sequence:
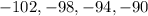
This is an arithmetic sequence that means that each term can be found by adding a constant term to the previous term. In this case, that constant term is 4, since:
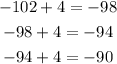
This term is called the common difference. The n-th term of an arithmetic sequence is given by:

Where:
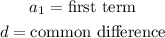
In this case, we have:
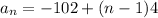
Solving the operations we get:
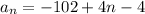
Simplifying:

Now we replace "n = 55" since we want to find the 55th term:
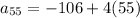
Solving the operations:
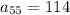
Therefore the 55th term is 114