Question

mim2F= Gd21. Calculate the force of gravity on a 1-kg mass at Earth'ssurface. The mass of Earth is 6.0 x 1024 kg, and its radiusis 6.4 X 106 m.2. Calculate the force of gravity on the same 1-kg mass if itwere 6.4 X 10 m above Earth's surface (that is, if it were[Wo Earth radii from Earth's center).3. Calculate the force of gravity between Earth (mass =6.0 X 1024 kg) and the Moon (mass = 7.4 X 1022 kg).The average Earth-Moon distance is 3.8 x 108 m.4. Calculate the force of gravity between Earth and the Sun(the Sun's mass = 2.0 X 10% kg average Earth-Sundistance = 1.5 X 10" m).5. Calculate the force of gravity between a newborn baby(mass = 3 kg) and the planet Mars (mass = 6.4 X102 kg) when Mars is at its closest to Earth (distance =5.6 x 1010 m).6. Calculate the force of gravity between a newborn baby ofmass 3 kg and the obstetrician of mass 100 kg, who is0.5 m from the baby. Which exerts more gravitationalforce on the baby, Mars or the obstetrician? By how much?

Answer 1

Given that the mass of the object is

`m_1=\text{ 1 kg}`

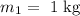
The mass of the earth is

The radius of the earth is

`d=6.4*10^6\text{ m}`

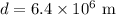
The value of gravitational constant

`G=6.67*10^(-11)Nkg^(-2)m^2`

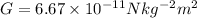
The formula to calculate gravitational force is

`F=(Gm_1m_2)/(d^2)`

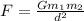
Substituting the values, the gravitational force will be

`\begin{gathered} F=(6.67*10^(-11)*1*6*10^(24))/((6.4*10^6)^2) \\ =9.77\text{ N} \end{gathered}`

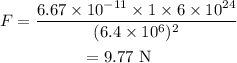
The gravitational force is 9.77 N