Question

Cassie has test grades of 62, 85 and 89 on the first three tests in herpre-algebra class. What are the possible scores she can make on thefourth test in order to make at least a letter grade of B after the fourthtest? A letter grade B means an average of at least 80. Let x represent thescore on the fourth test.(Write the answer in inequality form)

Answer 1

Step-by-step explanation:

The test grade for 1st three tests = 62, 85, 89

Let the fourth test score = x

The result we hoping to get is grade B.

Let's find the average of the four test score

`\text{Average = }\frac{Su\text{ m of all test score}}{nu\text{mber of test score}}`
`\begin{gathered} s\text{ um of test scores = 62 + 85 + 89 + x} \\ nu\text{mber of test score = 4} \\ \frac{\text{62 + 85 + 89 + x}}{4}=\text{ Average} \end{gathered}`
`\begin{gathered} A\text{ grade B represents atleast 80} \\ \text{Atleast represents }\ge \\ \frac{\text{62 + 85 + 89 + x}}{4}\text{ }\ge\text{ 80} \\ \frac{\text{236 + x}}{4}\text{ }\ge\text{ 80} \end{gathered}`
`\begin{gathered} \text{236 + x }\ge\text{ }80(4) \\ \text{236 + x }\ge\text{ }320 \\ \text{subtract }236\text{ from both sides:} \\ 236\text{ - 236 + x }\ge\text{ }320\text{ - 23}6 \\ x\text{ }\ge\text{ 84} \end{gathered}`

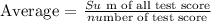
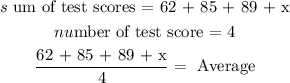
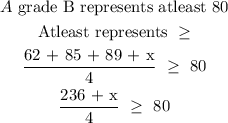
This means Cassie needs to score 84 or more to get a grade of B

In ineq