Question

Write the equation below in standard form and then answer the following questions. If a value is a non-integer type your answer as a reduced fraction. Type the center of the circle as the point (x,y) be sure to include parentheses and a comma between the values. 4x^2+y^2=4 The value for the denominator on x is: AnswerThe value for the denominator on y is: AnswerThe center is at the point Answer

Answer 1

Answer:(a) see equation below under point (a)

(b) The value for the denominator on x is: 1

(c) The value for the denominator on y is: 4

(d) The center is at the point = ( 0,0)

Explanations :

GIVEN :

4x^2 +y^2 = 4

This is of the form :

(x-a) ^2 =(y-b)^2 = r^2

where ( a;b) is the centere and r is the radius

The graph below show the x and y intercept and the centre of the graph 4x^2 +y^2 = 4

• This shows the centre ecclipses at (h;k) = (0;0) , b =2 and a = 1

(a) The standard form of the equation will be :

`\begin{gathered} ((x-h)^2)/(a^2)\text{ +}((y-k)^2)/(b^2) \\ \end{gathered}`

rewrite 4x^2 +y^2 = 4 in the standard ecclipse equation :

`((x-0)^2)/(1^2)+((y-0)^2)/(2^2)=\text{ 1}`

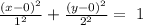
(b) The value for the denominator on x is: 1

(c) The value for the denominator on y is: 4

(d) The center is at the point = ( 0,0)